



Next: Galvez Topological Charge Indices[7,8,9]
Up: Molecular Descriptors
Previous: Autocorrelation Descriptors[1,13,12,11]
Contents
These descriptors are the eigenvalues of a modified connectivity
matrix, the Burden matrix 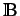 . The
. The 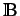 matrix is
an H depleted molecular graph defined as
matrix is
an H depleted molecular graph defined as
- diagonal elements are atomic numbers,
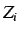 , of the
elements
, of the
elements
- off diagonal elements,
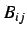 , representing bonded
atoms
, representing bonded
atoms 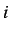 and
and 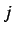 are equal to
are equal to
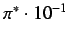 where
where 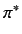 is the conventional bond order (ie 1,2,3,1.5 for single, double,
triple and aromatic bonds respectively)
is the conventional bond order (ie 1,2,3,1.5 for single, double,
triple and aromatic bonds respectively)
- Off diagonal elements corresponding to terminal bonds are
augmented by .01
- All other matrix elements are set to .001
The ordered sequence of the 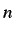 smallest eigenvalues of
smallest eigenvalues of 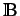 was proposed as a molecular descriptor based on the assumption that
the lowest eigenvalues contain contributions from all the atoms and
thus reflect topology of the molecule.
was proposed as a molecular descriptor based on the assumption that
the lowest eigenvalues contain contributions from all the atoms and
thus reflect topology of the molecule.
The BCUT descriptors are an extension of the Burden eigenvalues and
consider 3 classes of matrices whose diagonal elements correspond to
- atomic charge related values
- atomic polarizability related values
- atomic H bond abilities
A variety of definition have been used for the off diagonal terms
and both 2D and 3D approaches are considered. The highest and lowest
eigenvalues of these matrices have been shown to be discriminating
descriptors.




Next: Galvez Topological Charge Indices[7,8,9]
Up: Molecular Descriptors
Previous: Autocorrelation Descriptors[1,13,12,11]
Contents
2003-06-16